example
A sphere has a radius
6 inches. Find 1. its volume and 2. its surface area.
Solution
Step 1 is the same for both 1. and 2., so we will show it just once.
| Step 1. Read the problem. Draw the figure and label
it with the given information. |
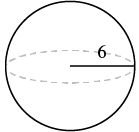 |
| 1. |
|
| Step 2. Identify what you are looking for. |
The volume of the sphere |
| Step 3. Name. Choose a variable to represent it. |
Let V = volume. |
| Step 4. Translate.
Write the appropriate formula. |
V=34πr3 |
| Step 5. Solve. |
V≈34(3.14)(6in)3
V≈904.32in3 |
| Step 6. Check: Double-check your math on a calculator. |
|
| Step 7. Answer the question. |
The volume is approximately 904.32 cubic inches. |
| 2. |
|
| Step 2. Identify what you are looking for. |
The surface area of the cube |
| Step 3. Name. Choose a variable to represent it. |
Let S = surface area. |
| Step 4. Translate.
Write the appropriate formula. |
S=4πr2 |
| Step 5. Solve. |
S≈4(3.14)(6in)2
S≈452.16in2 |
| Step 6. Check: Double-check your math on a calculator |
|
| Step 7. Answer the question. |
The surface area is approximately 452.16 square inches. |
example
A globe of Earth is in the shape of a sphere with radius
14 centimeters. Find 1. its volume and 2. its surface area. Round the answer to the nearest hundredth.
Answer:
Solution
| Step 1. Read the problem. Draw a figure with the
given information and label it. |
 |
| 1. |
|
| Step 2. Identify what you are looking for. |
The volume of the sphere |
| Step 3. Name. Choose a variable to represent it. |
Let V = volume. |
| Step 4. Translate.
Write the appropriate formula.
Substitute. (Use 3.14 for π ) |
V=34πr3
V≈34(3.14)(14cm)3 |
| Step 5. Solve. |
V≈11,488.21cm3 |
| Step 6. Check: We leave it to you to check your calculations. |
|
| Step 7. Answer the question. |
The volume is approximately 11,488.21 cubic centimeters. |
| 2. |
|
| Step 2. Identify what you are looking for. |
The surface area of the sphere |
| Step 3. Name. Choose a variable to represent it. |
Let S = surface area. |
| Step 4. Translate.
Write the appropriate formula.
Substitute. (Use 3.14 for π ) |
S=4πr2
S≈4(3.14)(14cm)2 |
| Step 5. Solve. |
S≈2461.76cm2 |
| Step 6. Check: We leave it to you to check your calculations. |
|
| Step 7. Answer the question. |
The surface area is approximately 2461.76 square centimeters. |
Did you have an idea for improving this content? We’d love your input.



